Lie group

Those geometries are themselves a Lie group $G$, so that the left action of $G$ on itself is an action by isometries.

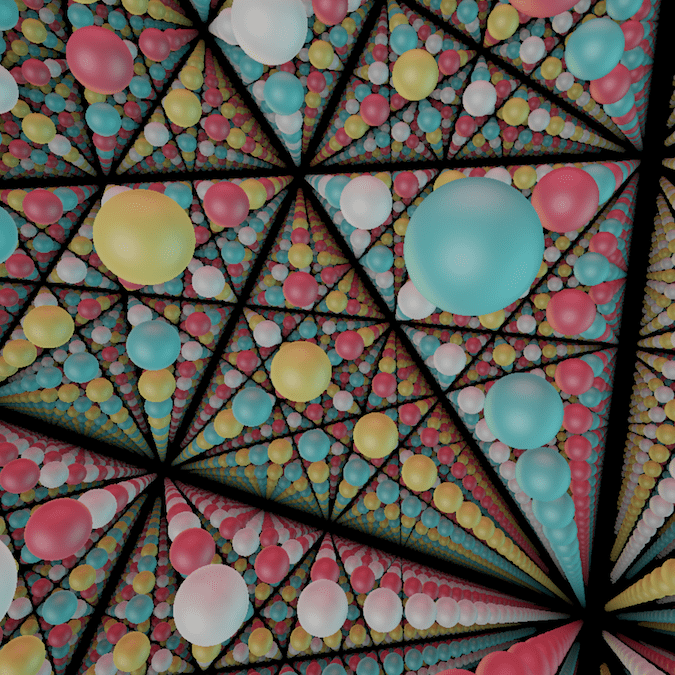
The euclidean space $\mathbb E^3$

The euclidean space corresponds to the “usual” 3D geometry we learn at school. It is the geometry of the flat real 3-dimensional vector space $\mathbb R^3$.

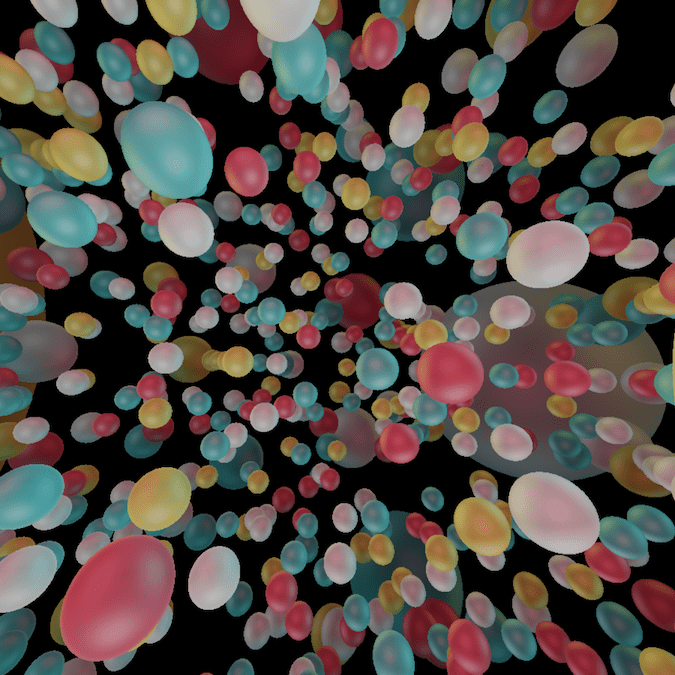
The spherical space $S^3$

The space $S^3$ is the 3-dimensional analogue of the usual sphere in 2 dimensions. It is an isotropic space (all the directions play the same role). Among the eight Thurston geometries, it is the only compact one. It can be seen as the unit tangent bundle of the 2-sphere. This point of view is also knwon as the Hopf fibration.

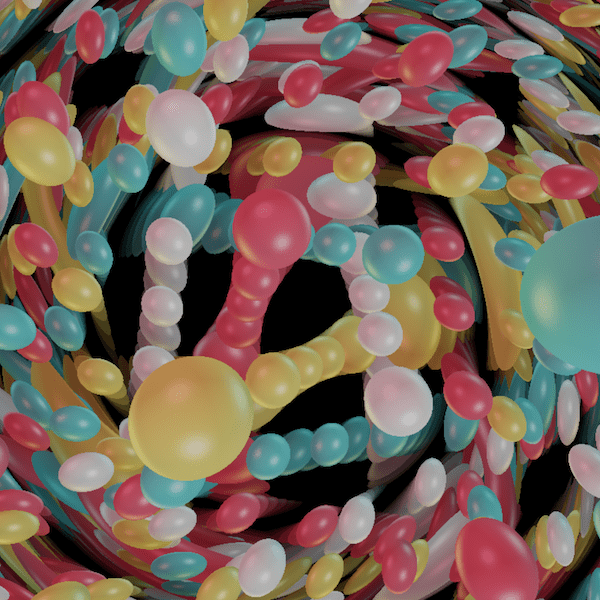
The Nil geometry

Nil is a 3-dimensional nilpotent Lie group. It can also be seen as the universal cover of the suspension of a 2-torus by a Dehn twist.

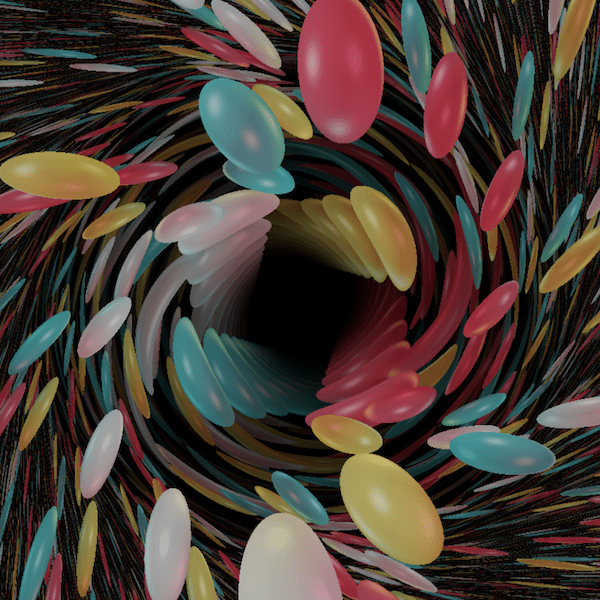
The universal cover of ${\rm SL}(2,\mathbb R)$

${\rm SL}(2,\mathbb R)$ is the set of all $2 \times 2$-real matrices with determinant one. The space $\widetilde{\rm SL}(2,\mathbb R)$ is its universal cover. There are several ways to think about this space. ${\rm SL}(2,\mathbb R)$ is for instance the unit tangent bundle of the hyperbolic plane $\mathbb H^2$. This point of view gives $\widetilde{\rm SL}(2,\mathbb R)$ a structure of a twisted metric line bundle over $\mathbb H^2$, which can be thought as a hyperbolic analogue of the Hopf fibration

The Sol geometry

Sol is a 3-dimensional solvable Lie group. It can also be seen as the universal cover of the suspension of a 2-torus by an Anosov matrix.